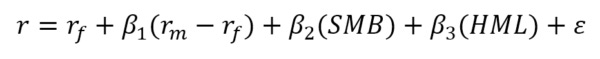Category: Investing Insight

Investing insight to make you a better investor.

Factors, Intro

First came the market, then came the factors…

The Efficient Market Hypothesis

Two economists walk down a road and they see a twenty dollar bill lying on the side-walk. One of them asks “is that a twenty dollar bill?” Then the other one answers “It can’t be, because someone would have picked it up already,” and they keep walking. (source)

In 1965, Eugene Fama published his dissertation arguing for the random walk hypothesis. i.e., stock market prices evolve according to a random walk (so price changes are random) and thus cannot be predicted. This was followed by Paul Samuelson, who published a proof showing that if the market is efficient, prices will exhibit random-walk behavior. (source)

Together, they form the basis of the efficient market hypothesis (EMH).

The efficient market hypothesis (EMH), is a hypothesis that states that share prices reflect all information and consistent alpha generation is impossible. According to the EMH, stocks always trade at their fair value on exchanges, making it impossible for investors to purchase undervalued stocks or sell stocks for inflated prices. Therefore, it should be impossible to outperform the overall market through expert stock selection or market timing, and the only way an investor can obtain higher returns is by purchasing riskier investments. (source)

Inefficiencies are opportunities

Any market practitioner knows that this is not entirely true. There are numerous hurdles in the way of pure efficiency:

  1. Information is not free.

  2. Liquidity is not unlimited.

  3. Prices are not continuous.

  4. Market statistics are forever in flux.

  5. Investors have different goals and pursue different outcomes.

  6. Taxes, rules and regulations.

According to EMH, a portfolio’s return could be fully explained by the market (source):

r = rf+ ß(rm – rf) + α

Where:

  • r = Expected rate of return

  • rf = Risk-free rate

  • ß = Beta

  • (rm – rf)= Market risk premium

This is a single-factor model. i.e., portfolio returns are only explained by market risk (rm – rf: market risk premium.) Whatever cannot be explained by the market is α, or the portfolio manager’s skill.

However, if you setup a portfolio in certain ways, you consistently ended up with higher returns, implying that there was something about the market, something systematic, that was not being captured by this equation. So, if you were rewarding a portfolio manager only on the basis of α calculated from the above equation, then you were probably over-paying the PM for harvesting something that the market offered for “free.”

In 1992, Eugene Fama and Kenneth French designed a model to fix this – the Fama–French three-factor model.

The Fama-French model aims to describe stock returns through three factors: (1) market risk (rm – rf: market risk premium,) (2) the outperformance of small-cap companies relative to large-cap companies (SMB: Small Minus Big,) and (3) the outperformance of high book-to-market value companies versus low book-to-market value companies (HML: High Minus Low.) The rationale behind the model is that high value and small-cap companies tend to regularly outperform the overall market. (source)

Where:

  • r= Expected rate of return

  • rf = Risk-free rate

  • ß = Factor’s coefficient (sensitivity)

  • (rm – rf)= Market risk premium

  • SMB(Small Minus Big) = Historic excess returns of small-cap companies over large-cap companies

  • HML(High Minus Low) = Historic excess returns of value stocks (high book-to-price ratio) over growth stocks (low book-to-price ratio)

  • ↋= Risk, or α

Think of SMB and HML as “base-rates.” A portfolio’s returns can now be explained by the degree of tilt (factor cofficients, ßs) it has towards value (HML) and small-caps (SMB). Furthermore, you can set up incentives for the portfolio manager that incorporates these factors so that he is rewarded only if he can out-perform a generic small-cap/value portfolio.

The academic definition of value (HML) and small-caps (SMB) is quite different from what investors are used to colloquially. Fama and French were interested in the decomposition of portfolio returns into sub-components (factors) and the persistence of these factors over many years/decades. They did this by constructing SMB and HML as long-short portfolios and analyzing the spread. It is very different from what the media refers to by these terms. It is useful to think of these as spreads and not as typical long-only “value” portfolio.

No pain, No gain

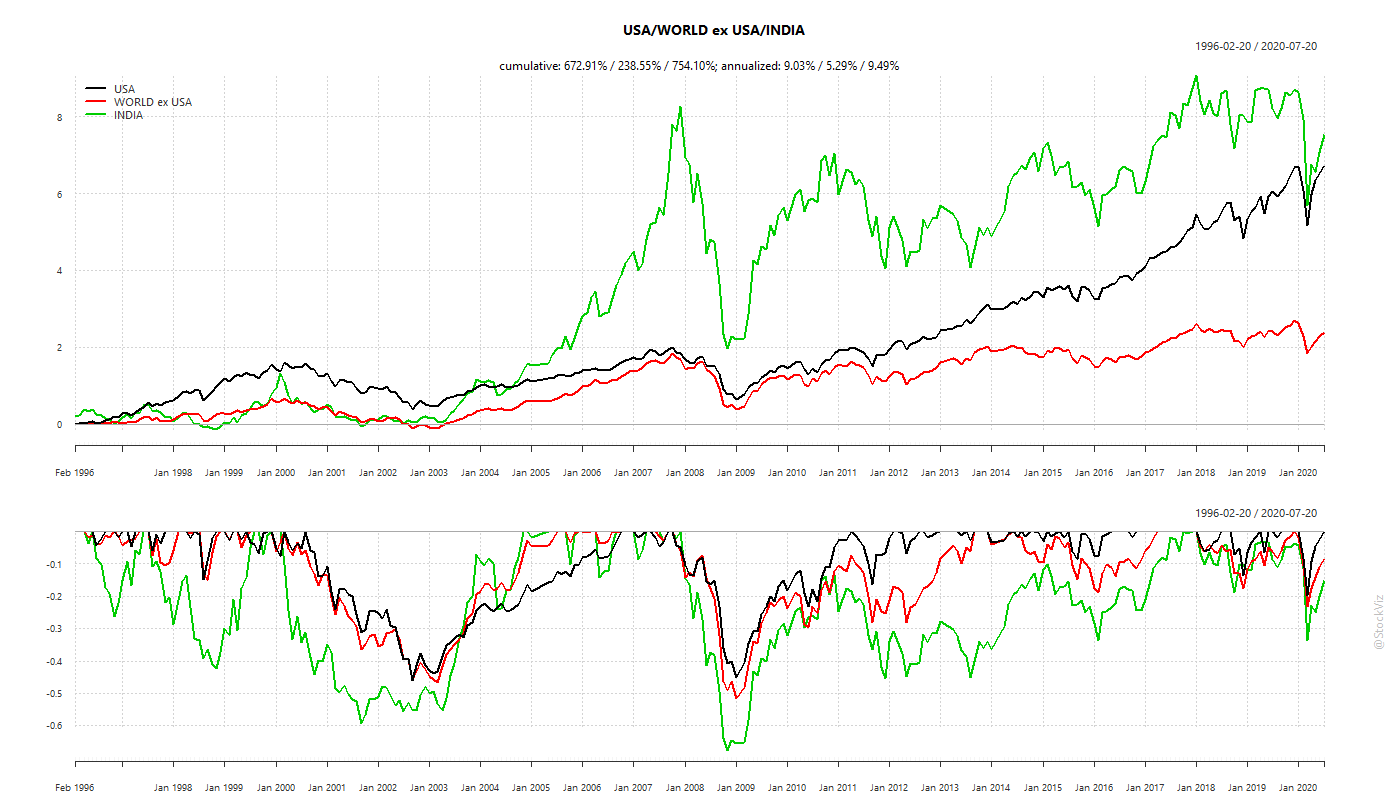
Just because these factors are persistent, doesn’t mean that they are always positive. A simple way to visualize this is to see the cumulative returns of the market risk premium (MKT = rm – rf) over SMB and HML.

There are periods of both out-performance and under-performance. In fact, one of the theories proposed to explain the persistence of these factors is behavioral: investors herd into value or small-caps based on recent out-performance. Thus, setting them up for subsequent under-performance. Upon which, they will exit en masse, allowing the factors to out-perform once again.

The real world is messy

Most investors are long-only. Whey we buy a value fund, for example, we are not really buying a Fama-French HML long-short portfolio. Our portfolios have a market beta and a bunch of other things affecting it besides high book-to-price ratio.

To visualize this, if we decompose the iShares Russell 1000 Value ETF, IWD, to its 3-factors, we get:

rf + 0.92*(rm – rf) – 0.06*SMB + 0.35*HML + α

Compared to iShares Russell 1000 Growth ETF, IWF:

rf + 1.03*(rm – rf) – 0.05*SMB – 0.27*HML + α

Conclusion

The Fama-French 3-Factor model is a useful tool to analyze investment portfolios. It allows us to decompose returns to different factors so that we can better understand the drivers of returns.

Coming up next: The Fama-French 5-Factor model.

Enjoy our discussion:

Categories Tags

Fat Tails, Everywhere

There is no asset free of extreme tail losses. If an asset produces any sort of return, it is going to be exposed to some sort of tail event.

One can try to find uncorrelated assets so that those losses don’t occur at the same time. However, correlations between asset returns are not stable – they change over time and behave quite erratically during market panics.

In the end, to be an investor is to accept the fact that large losses occasionally happen.

Categories Tags ,

Fat Tails, Expected Shortfall

No matter how you slice it, there is no escaping tail events in investing. It is the nature of the beast and every attempt you make eliminating the risk results in you giving up a significant portion of your returns. But given two investment opportunities, how do you go about figuring out which one is more susceptible to tail events?

Expected shortfall (ES) is a risk measure that can be used to estimate the loss during tail-events. The “expected shortfall at q% level” is the expected return on the portfolio in the worst q% of cases. ES estimates the risk of an investment in a conservative way, focusing on the less profitable outcomes. For high values of q it ignores the most profitable but unlikely possibilities, while for small values of q it focuses on the worst losses. Typically q is 5% and in formulae, p (= 100% – q) is often used as a substitute.

ES of Weekly Returns

Here’s a dilemma that most investors face: Mid-caps have given higher returns in the past compared to large-caps. But, how do their tail-risks compare?

Turns out, ES of the NIFTY MIDCAP 150 TR index is -6.73% vs. NIFTY 50 TR’s -5.65%. This is how much an investor would have lost in the worst 5% of weeks since 2011.

Sampling

In our previous post, we showed how strata-sampling can be used to make sure that you don’t end up ignoring tail-risk in your simulations. By definition, tail-events are rare. So, the differences are subtle.

Tactical Allocation

Reducing tail-risk is one of the biggest draws of tactical allocation. Anything that reduces deep drawdowns has the effect of keeping investors faithful to their investment process.

One way to setup a tactical allocation strategy is to use a Simple Moving Average (SMA) to decide between equity and bond allocations. Different SMA look-back periods will result in different levels of risk and reward. From an ES point of view, here’s how things for NIFTY shakes out:

Since 1999
Since 2010

Using an SMA and re-balancing weekly significantly reduces tail-risk.

How far back should you go?

The problem with tail-events is that there aren’t enough of them to build an effective model. There’s always a temptation to use as much data as possible so that these events find sufficient representation. However, markets evolve, regulatory structures change and past data stop being representative.

For example, if you run a tactical allocation back-test with all the data that is available, you’ll conclude that shorter the SMA, the better:

However, if you remove 2008 and its aftermath and look only are the data from 2011 onward, you get a different picture:

While metrics like ES and strategies like SMA are useful, the data that they are presented will give different results based on the regime that they are drawn from.

Risk management is a continuous process and cannot be reduced to single number.

Categories Tags ,

Save. Don't Invest.

Focus on what is important in life.

Often, the highest selling product is the one with the best narrative, not the one that provides the best value for money. Similarly, what drives eye-balls in media are narratives around “alpha,” “out-performance,” “best mutual funds,” “1% a day option strategies,” etc. But what really matters to you are things like “fund my kids’ college education,” “retire at a reasonable age,” “take a foreign vacation,” etc.

While it can be sexy to consider oneself as an investor – fantasizing belonging to the same tribe as Warren Buffett or Peter Lynch – people looking to meet goals that actually make a difference to their lives, are better off considering themselves as savers.

One saves their income to meet expenses in the future. Thinking this way drives focus towards the two things that are entirely within one’s control: savings rate and duration.

Once you shift the internal narrative to saving over investing, how you measure success changes as well. The last thing you’d want to do is play snakes-and-ladders with your portfolio. You’ll want to reduce risk as you get closer to withdrawal. And finally, you’ll realize that market’s return doesn’t matter as long as your funding needs are met.

Accepting lower returns

While you are stuck in Silk-Board traffic the next time, ponder this:

The current holder of the Outright World Land Speed Record is ThrustSSC driven by Andy Green, a twin turbofan jet-powered car which achieved 763.035 mph – 1227.985 km/h – over one mile in October 1997.

How come you don’t drive a jet-powered car? Wouldn’t that be the “best” car?

The reason why you drive a mini-van and not a jet is because your life involves mundane things like grocery shopping, dropping your kids off to school, going to the mall, etc.

Similarly, your portfolio should reflect your life. Just like you make-do with a mini-van (not the fastest, not the sexiest, but practical,) you should construct a portfolio that gets the job done. And that often involves not being the “best” investor but focusing on risk.

Managing risk means accepting that you will never go as fast as Andy Green.

Market returns vs. Portfolio returns

A “lost-decade” for stocks probably happens every decade.

Here’s MSCI indices for US, Developed Markets ex-US and India. The only thing that can be said for certain is that stocks fluctuate and occasionally tank 30-40% and sometimes even 70%

If you only invested in stocks and you needed money to send your kinds to college in 2008, then, well, good luck!

A goal-oriented portfolio constructed with this in mind will necessarily under-perform an all-stock portfolio when stocks are screaming higher. It will also not go down in flames when stocks tank, as they very often do. So, market returns ≠ portfolio returns.

Take risks when you can, not when you have to

If you are saving for a goal 10 years away, it is safer to bet that markets will recover in 10 years than to bet that they will recover in one. So it maybe a good idea to load-up on risk at the beginning and slowly de-risk as time goes on. In fact, if you don’t take risk upfront, then you maybe doing it wrong.

Here’s the difference between starting at 60% NIFTY vs. 95% and slowly ramping down over 10 years:

Glide path and Target-dates

The strategy described above is an example of a 10-year target-date fund with a linear glide-path. You start with an allocation that you are comfortable with – can be anything from 60/40 through 95/5 split between equities and bonds – and then every month nudge it so that at the “target-date” the allocation becomes 5/95. It is a way of taking risks upfront and de-risking the portfolio as the withdrawal date gets closer.

For longer time-horizons, say 30 years, you can also look at an exponential glide-path. The basic concept remains the same: reduce risk as you get closer towards withdrawal.

While decidedly unsexy, this “mini-van” strategy will safely get you and your family where you need to go.

Categories Tags

Fat Tails, Sampling

While developing a model, historical data alone may not be sufficient to test its robustness. One way to generate test data is to re-sample historical data. This “re-arrangement” of past time-series can then be fed to the model to see how it behaves.

The problem with sampling historical market data is that it may not sufficiently account for fat-tails. Typically, a uniform sample is taken. The problem with this is it under-represents the tails. This leads to models that work on average but blow up on occasion. Something you’d like to avoid.

One way to overcome this problem is through stratified sampling. You chop the data into intervals and use their frequencies to probability weight the sample. This preserves the original distribution in the sample.

Notice the skew and the tails in the “STRAT” densities for both NIFTY and MIDCAP indices. This distribution is far more likely to result in a robust model compared to the one that just uses uniform sampling.

You can check out the R-code here.

Categories Tags ,